Skip to main content

Section 3 Math 104 Problems

Students entering Math 104 should be able to successfuly complete the problems below.

Exercises Exercises

1.

Use the polynomial \(5x+2x^2y-4y\) to complete the following parts.

  1. Identify the constant and variables for the term with the largest degree in the polynomial.

  2. Given that \(x=4\) and \(y=-\dfrac{1}{4}\text{,}\) evaluate the polynomial expression.

Solution.

  1. The term with the largest degree is \(2x^2y\text{,}\) so the constant is \(2\) and the variables are \(x\) and \(y\text{.}\)

  2. \begin{align*} 5(4) + 2(4)^2\left(-\frac{1}{4}\right) - 4\left(-\frac{1}{4}\right) \amp = 20+2(16)\left(-\frac{1}{4}\right) + 1 \\ \amp = 20-8+1\\ \amp = 13 \end{align*}

2.

Consider the linear equation \(4x-3y+12=0\text{.}\)

  1. Solve the equation for \(y\text{.}\)

  2. Use the equation to complete the following table:
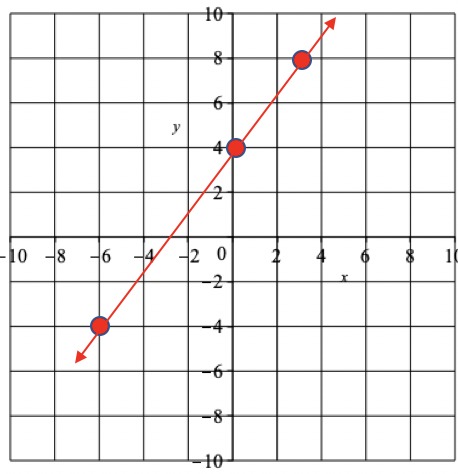
    \(x\) \(y\)
    \(-6\)
    \(4\)
    \(3\)

Give the coordinates of the points found in part b., and then plot the points and graph the linear equation.

Solution.

  1. \begin{gather*} -3y = -4x-12\\ y =\frac{4}{3}x + 4 \end{gather*}

  2. \(x\) \(y\)
    \(-6\) \(-4\)
    \(0\) \(4\)
    \(3\) \(8\)
  3. The points are \((-6,-4), (0,4)\text{,}\) and \((3,8)\text{.}\) The points and linear equation are graphed below.
3.

In a classroom of \(25\) students, \(3\) are left-handed and \(22\) are right-handed.

  1. What proportion of the class is left-handed?

  2. Write the proportion found in part a as a percentage.

  3. What is the ratio of left-handed students to right-handed students?

Solution.

  1. \(\displaystyle \dfrac{3}{25}\)

  2. \(\displaystyle 6\%\)

  3. \(\displaystyle 3: 22\)